Common Clinical Chemistry Tests

The tests listed below are conducted routinely by the Chemistry Section of the Clinical Pathology Laboratory in almost every hospital of greater than small size. 

                 TEST                     REFERENCE RANGE
             ---------------------        ---------------
             Sodium                        135-145 mEq/L
             Potassium                     3.5-5.5 mEq/L
             Chloride                      95-105  mEq/L
             Total CO2                     24-32   mmoles/L
             Glucose                       60-110  mg/dl
             Urea (BUN)                    10-20   mgN/dl
             Creatinine                    0.5-1.4 mg/dl
             Uric Acid                     2.5-8.0 mg/dl
             Inorganic Phosphate           2.3-4.8 mgP/dl
             Calcium                       8.5-10.8 mg/dl
             Total Protein                 6.0-8.0  g/dl
             Albumin                       3.5-5.0  g/dl
             Bilirubin                     0.1-1.4 mg/dl
             Cholesterol                   125-300 mg/dl 
             Triglycerides                    <170 mg/dl
             Alkaline Phosphatase           35-125 mU/ml
             Transaminases
                SGOT (AST)                   7-40  mU/ml
                SGPT (ALT)                   7-80  mU/ml
             Lactic Dehydrogenase (LDH)    105-215 mU/ml
             Creatine Phosphokinase (CPK)     <100 mU/ml
             Amylase                       Depends Upon Method
             Lipase                        Depends Upon Method
Results from these tests provide valuable clinical information about whether specific tissue has been damaged and how severely, and about the functional status of a variety of organ systems. The clinical significance of these tests, and others, is the major topic for the clinical pathology sessions throughout the course. Test results are interpreted with respect to reference ranges, which are, most typically, 95% confidence intervals of values found in clinically healthy populations.
Basic Statistical Considerations

Reference Ranges
Results are most typically interpreted with respect to the range of values found in normal, clinically healthy individuals. A result is considered abnormal if the value is less than the lower limit of the normal range or is greater than the upper limit. Normal ranges are determined from measurements on specimens from a large number (several hundred) of clinically normal individuals. When results are plotted in histogram fashion a distribution such as that illustrated below is obtained.

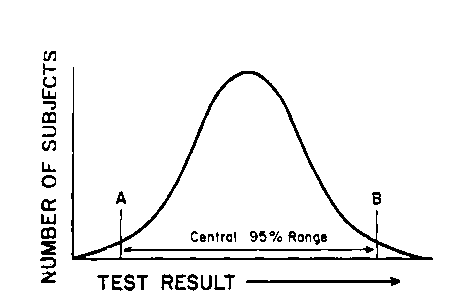
The normal range, or reference range, is determined by lower and upper limit values, as represented by test result values A and B in the figure to the right, which include 95% of all of the values. The distribution of values, in many cases, is Gaussian, bell-shaped, or uniform, as in the figure, and the normal range is relatively easily determined from the mean value and the standard deviation (S.D.), i.e.:

lower limit (A) = mean value - 2 S.D.
upper limit (B) = mean value + 2 S.D.
Not all test results from a clinically normal population distribute uniformally. Total bilirubin is an example and in such cases a more tedious, nonparametric procedure must be used to determine the lower and upper limits which include 95% of the population.
In some cases the upper and lower limits comprising 95% of a normal population is not the appropriate refence range. Total serum cholesterol is a case in which the usually quoted reference range is determined as a "healthy" range on the basis of results from long term epidemiologic studies, such as the Framingham study. In other cases, of which serum creatinine is an example, it is most appropriate to compare a current value to a previously determined value. Similarly, endocrinologic testing is often based upon comparing test results before and after administration of a stimulus or inhibitor. In general, pathology is often effectively evaluated on the basis of monitoring changes in laboratory values.

Precision
In monitoring a pathologic process on the basis of changing laboratory values, it must be recognized that laboratory results are determined with limited precision. Two laboratory test results can be interpreted reliably as different only if the two values differ by a minimum amount. The more precise a laboratory test result, the more significant is a small difference between two values.
Precision is generally expressed in relative terms as the coefficient of variation (CV). The coefficient of variation is determined by C.V. = 100 x S.D./mean. The CV of most laboratory tests is in the vicinity of 2 - 3%. The relative precision of enzyme determinations is generally poorer with C.V.s in the vicinity of 5%. The precision of Na+ determinations is considerably better with C.V.s generally in the vicinity of 1%.
The precision of individual laboratory tests is continuously monitored by including control specimens in every run of patient specimens. Control specimens are prepared as aliquots from a large volume of pooled serum which can be analyzed for as long as a year. As the control specimens are repeatedly analyzed, the mean and standard deviation are calculated, as is the C.V.

The significance of expressing precision in relative terms is illustrated by considering how to approach answering the following question.
What is the 95% confidence interval for a glucose result reported as 250 mg/dl?

You ask the laboratory to inform you about the precision of the glucose method in use. You are told that 2 control specimens, C1 and C2, are routinely measured each day and that their 95% confidence interval has consistently been 95 - 105 mg/dl and 332 - 367 mg/dl, respectively.
So you wonder how to translate this information to be relevant in answering your question about the precision associated with a result of 250 mg/dl, which is considerably different from the mean values of the control specimens, 100 and 350 mg/dl, respectively.
Considering the relative precision of the two control results provides the means for the translation to answer your question:

for C1:      CV = 100x[(105 - 95)/4]/100 = 2.5%
for C2:      CV = 100x[(367 - 332)/4]/350 = 2.5%

The relative precision of 2.5% can be applied to a result of any value and when applied to your result of 250 mg/dl, we find that:
1 SD = .025 x 250 mg/dl = 6.25 mg/dl.

The 95% confidence interval spans 4 SD, ie, 250 ± 12.5 and is therefor 237 - 262 mg/dl for the value of 250 mg/dl.

Two laboratory results (X1 and X2) may be interpreted as different, at the 95% confidence level, when:
            100*|(X1 - X2)|/ X1 > 2 C.V.
 Predictive Values
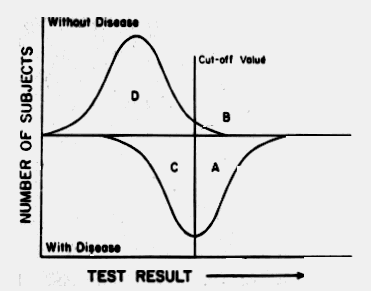
For some laboratory tests there is significant overlap between normal values and values from patients with disease, as illustrated below. In such cases the probability is limited that a laboratory test result will correctly diagnose or rule out disease.
Predictive values quantify the probability that a medical decision, based upon a laboratory test result, is correct. The three predictive values are:
  • P.V.P.= Predictive Value of a Positive test result
  • P.V.N.= Predictive Value of a Negative test result
  • Eff. = Combined accuracy of both positive and negative test results

    The probability quantified by each predictive value is described by the following:
  • PVP is the probability that an abnormal (positive) test result is associated with disease.
  • PVN is the probability that a normal test result is not associated with disease.
  • Eff is the total probability for the accuracy of both normal and abnormal test results.

    • Determination of predictive values requires knowledge of each of the following:
  • prevalence of the disease in the group to be examined,
  • sensitivity of the test and
  • specificity of the test.

    Each of the above may be determined experimentally and are defined by:
  • Prevalence = fraction of diseased subjects in the total population = number of diseased / total population
  • Sensitivity = fraction of true positives = number of true positives / total number of patients with disease {= A/(A+C) in the above}
  • Specificity = fraction of true negatives = number of true negatives / total number of healthy individuals {= D/(D+B) in the above}

    • Determination of the three predictive values follows§ from the definiton of each:

    P.V.P.= Predictive Value of a Positive test result
      = number of true positives /(true positives+false positives)
      = TP / ( TP + FP )                          
      = prevalence*sensitivity/[prevalence*sensitivity+(1-prevalence)*(1-specificity)]
        
P.V.N.= Predictive Value of a Negative test result
      = number of true negatives /(true negatives + false negatives)
      = TN / ( TN + FN )
      = (1-prevalence)*specificity/[(1-prevalence)*specificity+prevalence*(1-sensitivity)]  
  
 Eff. = Efficiency                                                       
      = (true positives + true negative)/(total healthy + total diseased)
      = ( TP + TN )/( TP + FN + TN + FP )
      = [prevalence*sensitivity + (1-prevalence)*specificity]/ 1
    § Note that:
    fraction diseased = prevalence
    fraction healthy = 1 - prevalence
    true positives = (fraction diseased)*sensitivity = prevalence*sensitivity
    false negatives = prevalence*(1-sensitivity)
    true negatives = (fraction healthy)*specificity = (1-prevalence)*specicicity
    false positives = (1-prevalence)*(1-specificity)

    Consider an example

    Suppose:
    A new test for detecting rheumatoid arthritis is developed. Initial studies show that the test is positive in 99 of a group of 100 subjects known to have the disease. The sensitivity of the test is 99%.
    100 subjects, known to not have the disease, are tested and only one has a positive result. The specificity of the test is 99%.
    If the prevalence of R.A. is 1 per 100 of the local, general population who are likely to be tested, then:

    P.V.P. = T.P. / ( T.P. + F.P. ) = .0099 / (0.0099 + 0.0099) = 50 %
    P.V.N. = T.N. / ( T.N.+ F.N. ) = 0.98 / (0.98 + 0.0001 ) = 100 %
    Eff. = (T.P. + T.N. ) / 1 = (.0099 + 0.98 ) / ( 1 ) = 99%